The reciprocal of the decimal logarithm. Definition of the logarithm and its properties: theory and problem solving

In ratio

the task of finding any of the three numbers from the other two given ones can be set. If a and then N are given, they are found by exponentiation. If N and then a are given by taking the root of the degree x (or raising it to the power). Now consider the case when, given a and N, we need to find x.

Let the number N be positive: the number a be positive and not equal to one: .

Definition. The logarithm of the number N to the base a is the exponent to which a must be raised to obtain the number N; logarithm is denoted by

Thus, in equality (26.1) the exponent is found as the logarithm of N to base a. Posts

have the same meaning. Equality (26.1) is sometimes called the main identity of the theory of logarithms; in reality it expresses the definition of the concept of logarithm. By this definition The base of the logarithm a is always positive and different from unity; the logarithmic number N is positive. Negative numbers and zero have no logarithms. It can be proven that any number with a given base has a well-defined logarithm. Therefore equality entails . Note that the condition is essential here; otherwise, the conclusion would not be justified, since the equality is true for any values of x and y.

Example 1. Find

Solution. To obtain a number, you must raise the base 2 to the power Therefore.

You can make notes when solving such examples in the following form:

Example 2. Find .

Solution. We have

In examples 1 and 2, we easily found the desired logarithm by representing the logarithm number as a power of the base with a rational exponent. In the general case, for example, for etc., this cannot be done, since the logarithm has an irrational value. Let us pay attention to one issue related to this statement. In paragraph 12 we gave the concept of the possibility of determining any real degree of a given positive number. This was necessary for the introduction of logarithms, which, generally speaking, can be irrational numbers.

Let's look at some properties of logarithms.

Property 1. If the number and base are equal, then the logarithm is equal to one, and, conversely, if the logarithm is equal to one, then the number and base are equal.

Proof. Let By the definition of a logarithm we have and whence

Conversely, let Then by definition

Property 2. The logarithm of one to any base is equal to zero.

Proof. By definition of a logarithm (the zero power of any positive base is equal to one, see (10.1)). From here

Q.E.D.

The converse statement is also true: if , then N = 1. Indeed, we have .

Before formulating the next property of logarithms, let us agree to say that two numbers a and b lie on the same side of the third number c if they are both greater than c or less than c. If one of these numbers is greater than c, and the other is less than c, then we will say that they lie along different sides from the village

Property 3. If the number and base lie on the same side of one, then the logarithm is positive; If the number and base lie on opposite sides of one, then the logarithm is negative.

The proof of property 3 is based on the fact that the power of a is greater than one if the base is greater than one and the exponent is positive or the base is less than one and the exponent is negative. A power is less than one if the base is greater than one and the exponent is negative or the base is less than one and the exponent is positive.

There are four cases to consider:

We will limit ourselves to analyzing the first of them; the reader will consider the rest on his own.

Let then in equality the exponent can be neither negative nor equal to zero, therefore, it is positive, i.e., as required to be proved.

Example 3. Find out which of the logarithms below are positive and which are negative:

Solution, a) since the number 15 and the base 12 are located on the same side of one;

b) since 1000 and 2 are located on one side of the unit; in this case, it is not important that the base is greater than the logarithmic number;

c) since 3.1 and 0.8 lie on opposite sides of unity;

G) ; Why?

d) ; Why?

The following properties 4-6 are often called the rules of logarithmation: they allow, knowing the logarithms of some numbers, to find the logarithms of their product, quotient, and degree of each of them.

Property 4 (product logarithm rule). Logarithm of the product of several positive numbers to a given base equal to the sum logarithms of these numbers to the same base.

Proof. Let the given numbers be positive.

For the logarithm of their product, we write the equality (26.1) that defines the logarithm:

From here we will find

Comparing the exponents of the first and last expressions, we obtain the required equality:

Note that the condition is essential; logarithm of the product of two negative numbers makes sense, but in this case we get

In general, if the product of several factors is positive, then its logarithm is equal to the sum of the logarithms of the absolute values of these factors.

Property 5 (rule for taking logarithms of quotients). The logarithm of a quotient of positive numbers is equal to the difference between the logarithms of the dividend and the divisor, taken to the same base. Proof. We consistently find

Q.E.D.

Property 6 (power logarithm rule). Logarithm of the power of some positive number equal to the logarithm this number multiplied by the exponent.

Proof. Let us write again the main identity (26.1) for the number:

Q.E.D.

Consequence. The logarithm of a root of a positive number is equal to the logarithm of the radical divided by the exponent of the root:

The validity of this corollary can be proven by imagining how and using property 6.

Example 4. Take logarithm to base a:

a) (it is assumed that all values b, c, d, e are positive);

b) (it is assumed that ).

Solution, a) It is convenient to go to fractional powers in this expression:

Based on equalities (26.5)-(26.7), we can now write:

We notice that simpler operations are performed on the logarithms of numbers than on the numbers themselves: when multiplying numbers, their logarithms are added, when dividing, they are subtracted, etc.

That is why logarithms are used in computing practice (see paragraph 29).

The inverse action of logarithm is called potentiation, namely: potentiation is the action by which the number itself is found from a given logarithm of a number. Essentially, potentiation is not special action: it comes down to raising the base to a power ( equal to the logarithm numbers). The term "potentiation" can be considered synonymous with the term "exponentiation".

When potentiating, one must use the rules inverse to the rules of logarithmation: replace the sum of logarithms with the logarithm of the product, the difference of logarithms with the logarithm of the quotient, etc. In particular, if there is a factor in front of the sign of the logarithm, then during potentiation it must be transferred to the exponent degrees under the sign of the logarithm.

Example 5. Find N if it is known that

Solution. In connection with the just stated rule of potentiation, we will transfer the factors 2/3 and 1/3 standing in front of the signs of logarithms on the right side of this equality into exponents under the signs of these logarithms; we get

Now we replace the difference of logarithms with the logarithm of the quotient:

to obtain the last fraction in this chain of equalities, we freed the previous fraction from irrationality in the denominator (clause 25).

Property 7. If the base is greater than one, then larger number has a larger logarithm (and a smaller number has a smaller one), if the base is less than one, then the larger number has a smaller logarithm (and the smaller number has a larger one).

This property is also formulated as a rule for taking logarithms of inequalities, both sides of which are positive:

When logarithming inequalities to a base greater than one, the sign of inequality is preserved, and when logarithming to a base less than one, the sign of inequality changes to the opposite (see also paragraph 80).

The proof is based on properties 5 and 3. Consider the case when If , then and, taking logarithms, we obtain

(a and N/M lie on the same side of unity). From here

Case a follows, the reader will figure it out on his own.

So, we have powers of two. If you take the number from the bottom line, you can easily find the power to which you will have to raise two to get this number. For example, to get 16, you need to raise two to the fourth power. And to get 64, you need to raise two to the sixth power. This can be seen from the table.

And now - actually, the definition of the logarithm:

The base a logarithm of x is the power to which a must be raised to get x.

Designation: log a x = b, where a is the base, x is the argument, b is what the logarithm is actually equal to.

For example, 2 3 = 8 ⇒ log 2 8 = 3 (the base 2 logarithm of 8 is three because 2 3 = 8). With the same success log 2 64 = 6, since 2 6 = 64.

The operation of finding the logarithm of a number to a given base is called logarithmization. So, let's add a new line to our table:

2 1	2 2	2 3	2 4	2 5	2 6
2	4	8	16	32	64
log 2 2 = 1	log 2 4 = 2	log 2 8 = 3	log 2 16 = 4	log 2 32 = 5	log 2 64 = 6

Unfortunately, not all logarithms are calculated so easily. For example, try finding log 2 5 . The number 5 is not in the table, but logic dictates that the logarithm will lie somewhere on the segment. Because 2 2< 5 < 2 3 , а чем more degree twos, the larger the number.

Such numbers are called irrational: the numbers after the decimal point can be written ad infinitum, and they are never repeated. If the logarithm turns out to be irrational, it is better to leave it that way: log 2 5, log 3 8, log 5 100.

It is important to understand that a logarithm is an expression with two variables (the base and the argument). At first, many people confuse where the basis is and where the argument is. To avoid annoying misunderstandings, just look at the picture:

Before us is nothing more than the definition of a logarithm. Remember: logarithm is a power, into which the base must be built in order to obtain an argument. It is the base that is raised to a power - it is highlighted in red in the picture. It turns out that the base is always at the bottom! I tell my students this wonderful rule at the very first lesson - and no confusion arises.

We've figured out the definition - all that's left is to learn how to count logarithms, i.e. get rid of the "log" sign. To begin with, we note that two important facts follow from the definition:

The argument and the base must always be greater than zero. This follows from the definition of a degree by a rational exponent, to which the definition of a logarithm is reduced.
The base must be different from one, since one to any degree still remains one. Because of this, the question “to what power must one be raised to get two” is meaningless. There is no such degree!

Such restrictions are called region acceptable values (ODZ). It turns out that the ODZ of the logarithm looks like this: log a x = b ⇒ x > 0, a > 0, a ≠ 1.

Note that there are no restrictions on the number b (the value of the logarithm). For example, the logarithm may well be negative: log 2 0.5 = −1, because 0.5 = 2 −1.

However, now we are considering only numerical expressions, where it is not required to know the VA of the logarithm. All restrictions have already been taken into account by the authors of the problems. But when logarithmic equations and inequalities come into play, DL requirements will become mandatory. After all, the basis and argument may contain very strong constructions that do not necessarily correspond to the above restrictions.

Now let's look at the general scheme for calculating logarithms. It consists of three steps:

Express the base a and the argument x as a power with the minimum possible base greater than one. Along the way, it’s better to get rid of decimals;
Solve the equation for variable b: x = a b ;
The resulting number b will be the answer.

That's it! If the logarithm turns out to be irrational, this will be visible already in the first step. The requirement that the base be greater than one is very important: this reduces the likelihood of error and greatly simplifies the calculations. It’s the same with decimal fractions: if you immediately convert them into ordinary ones, there will be many fewer errors.

Let's see how this scheme works using specific examples:

Task. Calculate the logarithm: log 5 25

Let's imagine the base and argument as a power of five: 5 = 5 1 ; 25 = 5 2 ;

Let's create and solve the equation:
log 5 25 = b ⇒ (5 1) b = 5 2 ⇒ 5 b = 5 2 ⇒ b = 2 ;

We received the answer: 2.

Task. Calculate the logarithm:

Task. Calculate the logarithm: log 4 64

Let's imagine the base and argument as a power of two: 4 = 2 2 ; 64 = 2 6 ;
Let's create and solve the equation:
log 4 64 = b ⇒ (2 2) b = 2 6 ⇒ 2 2b = 2 6 ⇒ 2b = 6 ⇒ b = 3 ;
We received the answer: 3.

Task. Calculate the logarithm: log 16 1

Let's imagine the base and argument as a power of two: 16 = 2 4 ; 1 = 2 0 ;
Let's create and solve the equation:
log 16 1 = b ⇒ (2 4) b = 2 0 ⇒ 2 4b = 2 0 ⇒ 4b = 0 ⇒ b = 0 ;
We received the answer: 0.

Task. Calculate the logarithm: log 7 14

Let's imagine the base and argument as a power of seven: 7 = 7 1 ; 14 cannot be represented as a power of seven, since 7 1< 14 < 7 2 ;
From the previous paragraph it follows that the logarithm does not count;
The answer is no change: log 7 14.

A small note on the last example. How can you be sure that a number is not an exact power of another number? It’s very simple - just factor it into prime factors. If the expansion has at least two different factors, the number is not an exact power.

Task. Find out whether the numbers are exact powers: 8; 48; 81; 35; 14.

8 = 2 · 2 · 2 = 2 3 - exact degree, because there is only one multiplier;
48 = 6 · 8 = 3 · 2 · 2 · 2 · 2 = 3 · 2 4 - is not an exact power, since there are two factors: 3 and 2;
81 = 9 · 9 = 3 · 3 · 3 · 3 = 3 4 - exact degree;
35 = 7 · 5 - again not an exact power;
14 = 7 · 2 - again not an exact degree;

Let us also note that we ourselves prime numbers are always exact degrees of themselves.

Decimal logarithm

Some logarithms are so common that they have a special name and symbol.

The decimal logarithm of x is the logarithm to base 10, i.e. The power to which the number 10 must be raised to obtain the number x. Designation: lg x.

For example, log 10 = 1; lg 100 = 2; lg 1000 = 3 - etc.

From now on, when a phrase like “Find lg 0.01” appears in a textbook, know: this is not a typo. This is a decimal logarithm. However, if you are unfamiliar with this notation, you can always rewrite it:
log x = log 10 x

Everything that is true for ordinary logarithms is also true for decimal logarithms.

Natural logarithm

There is another logarithm that has its own designation. In some ways, it's even more important than decimal. It's about about the natural logarithm.

The natural logarithm of x is the logarithm to base e, i.e. the power to which the number e must be raised to obtain the number x. Designation: ln x .

Many will ask: what is the number e? This is an irrational number, its exact value impossible to find and record. I will give only the first figures:
e = 2.718281828459...

We won’t go into detail about what this number is and why it is needed. Just remember that e is the base of the natural logarithm:
ln x = log e x

Thus ln e = 1 ; ln e 2 = 2; ln e 16 = 16 - etc. On the other hand, ln 2 is an irrational number. In general, the natural logarithm of any rational number irrational. Except, of course, for one: ln 1 = 0.

For natural logarithms, all the rules that are true for ordinary logarithms are valid.

Logarithms, like any numbers, can be added, subtracted and transformed in every way. But since logarithms are not exactly ordinary numbers, there are rules here, which are called main properties.

You definitely need to know these rules - without them, not a single serious logarithmic problem can be solved. In addition, there are very few of them - you can learn everything in one day. So let's get started.

Adding and subtracting logarithms

Consider two logarithms with the same bases: log a x and log a y. Then they can be added and subtracted, and:

log a x+ log a y=log a (x · y);
log a x− log a y=log a (x : y).

So, the sum of logarithms is equal to the logarithm of the product, and the difference is equal to the logarithm of the quotient. Please note: key point Here - identical grounds. If the reasons are different, these rules do not work!

These formulas will help you calculate logarithmic expression even when its individual parts are not counted (see lesson “What is a logarithm”). Take a look at the examples and see:

Log 6 4 + log 6 9.

Since logarithms have the same bases, we use the sum formula:
log 6 4 + log 6 9 = log 6 (4 9) = log 6 36 = 2.

Task. Find the value of the expression: log 2 48 − log 2 3.

The bases are the same, we use the difference formula:
log 2 48 − log 2 3 = log 2 (48: 3) = log 2 16 = 4.

Task. Find the value of the expression: log 3 135 − log 3 5.

Again the bases are the same, so we have:
log 3 135 − log 3 5 = log 3 (135: 5) = log 3 27 = 3.

As you can see, the original expressions are made up of “bad” logarithms, which are not calculated separately. But after the transformations, completely normal numbers are obtained. Many are built on this fact tests. Yes, test-like expressions are offered in all seriousness (sometimes with virtually no changes) on the Unified State Examination.

Extracting the exponent from the logarithm

Now let's complicate the task a little. What if the base or argument of a logarithm is a power? Then the exponent of this degree can be taken out of the sign of the logarithm according to the following rules:

It is easy to see that the last rule follows the first two. But it’s better to remember it anyway - in some cases it will significantly reduce the amount of calculations.

Of course, all these rules make sense if the ODZ of the logarithm is observed: a > 0, a ≠ 1, x> 0. And one more thing: learn to apply all formulas not only from left to right, but also vice versa, i.e. You can enter the numbers before the logarithm sign into the logarithm itself. This is what is most often required.

Task. Find the value of the expression: log 7 49 6 .

Let's get rid of the degree in the argument using the first formula:
log 7 49 6 = 6 log 7 49 = 6 2 = 12

Task. Find the meaning of the expression:
[Caption for the picture]

Note that the denominator contains a logarithm, the base and argument of which are exact powers: 16 = 2 4 ; 49 = 7 2. We have:

[Caption for the picture]

I think the last example requires some clarification. Where have logarithms gone? Until the very last moment we work only with the denominator. We presented the base and argument of the logarithm standing there in the form of powers and took out the exponents - we got a “three-story” fraction.

Now let's look at the main fraction. The numerator and denominator contain the same number: log 2 7. Since log 2 7 ≠ 0, we can reduce the fraction - 2/4 will remain in the denominator. According to the rules of arithmetic, the four can be transferred to the numerator, which is what was done. The result was the answer: 2.

Transition to a new foundation

Speaking about the rules for adding and subtracting logarithms, I specifically emphasized that they only work with the same bases. What if the reasons are different? What if they are not exact powers of the same number?

Formulas for transition to a new foundation come to the rescue. Let us formulate them in the form of a theorem:

Let it be given logarithm log a x. Then for any number c such that c> 0 and c≠ 1, the equality is true:
[Caption for the picture]
In particular, if we put c = x, we get:
[Caption for the picture]

From the second formula it follows that the base and argument of the logarithm can be swapped, but in this case the entire expression is “turned over”, i.e. the logarithm appears in the denominator.

These formulas are rarely found in conventional numerical expressions. It is possible to evaluate how convenient they are only when solving logarithmic equations and inequalities.

However, there are problems that cannot be solved at all except by moving to a new foundation. Let's look at a couple of these:

Task. Find the value of the expression: log 5 16 log 2 25.

Note that the arguments of both logarithms contain exact powers. Let's take out the indicators: log 5 16 = log 5 2 4 = 4log 5 2; log 2 25 = log 2 5 2 = 2log 2 5;

Now let’s “reverse” the second logarithm:

[Caption for the picture]

Since the product does not change when rearranging factors, we calmly multiplied four and two, and then dealt with logarithms.

Task. Find the value of the expression: log 9 100 lg 3.

The base and argument of the first logarithm are exact powers. Let's write this down and get rid of the indicators:

[Caption for the picture]

Now let's get rid of the decimal logarithm by moving to a new base:

[Caption for the picture]

Basic logarithmic identity

Often in the solution process it is necessary to represent a number as a logarithm to a given base. In this case, the following formulas will help us:

In the first case, the number n becomes an indicator of the degree standing in the argument. Number n can be absolutely anything, because it’s just a logarithm value.

The second formula is actually a paraphrased definition. That’s what it’s called: the basic logarithmic identity.

In fact, what will happen if the number b raise to such a power that the number b to this power gives the number a? That's right: you get this same number a. Read this paragraph carefully again - many people get stuck on it.

Like formulas for moving to a new base, the basic logarithmic identity is sometimes the only possible solution.

Task. Find the meaning of the expression:
[Caption for the picture]

Note that log 25 64 = log 5 8 - simply took the square from the base and argument of the logarithm. Taking into account the rules for multiplying powers with the same base, we get:

[Caption for the picture]

If anyone doesn't know, this was a real task from the Unified State Exam :)

Logarithmic unit and logarithmic zero

In conclusion, I will give two identities that can hardly be called properties - rather, they are consequences of the definition of the logarithm. They constantly appear in problems and, surprisingly, create problems even for “advanced” students.

log a a= 1 is a logarithmic unit. Remember once and for all: logarithm to any base a from this very base is equal to one.
log a 1 = 0 is logarithmic zero. Base a can be anything, but if the argument contains one, the logarithm is equal to zero! Because a 0 = 1 is a direct consequence of the definition.

That's all the properties. Be sure to practice putting them into practice! Download the cheat sheet at the beginning of the lesson, print it out, and solve the problems.

Logarithmic expressions, solving examples. In this article we will look at problems related to solving logarithms. The tasks ask the question of finding the meaning of an expression. It should be noted that the concept of logarithm is used in many tasks and understanding its meaning is extremely important. As for the Unified State Exam, the logarithm is used when solving equations, in applied problems, and also in tasks related to the study of functions.

Let us give examples to understand the very meaning of the logarithm:

Basic logarithmic identity:

Properties of logarithms that must always be remembered:

*The logarithm of the product is equal to the sum of the logarithms of the factors.

* * *

*The logarithm of a quotient (fraction) is equal to the difference between the logarithms of the factors.

* * *

*Logarithm of degree equal to the product exponent by the logarithm of its base.

* * *

*Transition to a new foundation

* * *

More properties:

* * *

The calculation of logarithms is closely related to the use of properties of exponents.

Let's list some of them:

The essence of this property lies in the fact that when transferring the numerator to the denominator and vice versa, the sign of the exponent changes to the opposite. For example:

A corollary from this property:

* * *

When raising a power to a power, the base remains the same, but the exponents are multiplied.

* * *

As you have seen, the concept of a logarithm itself is simple. The main thing is that you need good practice, which gives you a certain skill. Of course, knowledge of formulas is required. If the skill in converting elementary logarithms has not been developed, then when solving simple tasks you can easily make a mistake.

Practice, solve the simplest examples from the mathematics course first, then move on to more complex ones. In the future, I will definitely show how “ugly” logarithms are solved; these won’t appear on the Unified State Examination, but they are of interest, don’t miss them!

That's all! Good luck to you!

Sincerely, Alexander Krutitskikh

P.S: I would be grateful if you tell me about the site on social networks.

Definition of logarithm

The logarithm of b to base a is the exponent to which a must be raised to obtain b.

Number e in mathematics it is customary to denote the limit to which an expression strives

Number e is irrational number- a number incommensurable with one, it cannot be accurately expressed as either an integer or a fraction rational number.

Letter e- first letter Latin word exponere- to show off, hence the name in mathematics exponential- exponential function.

Number e widely used in mathematics, and in all sciences that in one way or another use mathematical calculations for their needs.

Logarithms. Properties of logarithms

Definition: The logarithm of a positive number b to its base is the exponent c to which the number a must be raised to obtain the number b.

Basic logarithmic identity:

7) Formula for moving to a new base:

lna = log e a, e ≈ 2.718…

Problems and tests on the topic “Logarithms. Properties of logarithms"

Logarithms - Important Topics for repeating the Unified State Examination in mathematics

To successfully complete tasks on this topic, you must know the definition of a logarithm, the properties of logarithms, the basic logarithmic identity, the definitions of decimal and natural logarithms. The main types of problems on this topic are problems involving the calculation and transformation of logarithmic expressions. Let's consider their solution using the following examples.

Solution: Using the properties of logarithms, we get

Solution: Using the properties of degrees, we get

1) (2 2) log 2 5 =(2 log 2 5) 2 =5 2 =25

Properties of logarithms, formulations and proofs.

Logarithms have a number of characteristic properties. In this article we will look at the main properties of logarithms. Here we will give their formulations, write the properties of logarithms in the form of formulas, show examples of their application, and also provide proof of the properties of logarithms.

Page navigation.

Basic properties of logarithms, formulas

For ease of remembering and use, let's imagine basic properties of logarithms in the form of a list of formulas. IN next point We will give their formulations, evidence, examples of use and necessary explanations.

Property of the logarithm of unity: log a 1=0 for any a>0, a≠1.

Logarithm of a number equal to the base: log a a=1 for a>0, a≠1.

Property of the logarithm of the power of the base: log a a p =p, where a>0, a≠1 and p is any real number.

Logarithm of the product of two positive numbers: log a (x y)=log a x+log a y , a>0 , a≠1 , x>0 , y>0 ,
and the property of the logarithm of the product of n positive numbers: log a (x 1 · x 2 ·…·x n)= log a x 1 +log a x 2 +…+log a x n , a>0 , a≠1 , x 1 >0, x 2 >0, …, x n >0 .

Property of the logarithm of a quotient:

, where a>0, a≠1, x>0, y>0.

Logarithm of the power of a number: log a b p =p·log a |b| , where a>0, a≠1, b and p are numbers such that the degree b p makes sense and b p >0.

Consequence:

, where a>0, a≠1, n – natural number, greater than one, b>0.

Corollary 1:

, a>0 , a≠1 , b>0 , b≠1 .

Corollary 2:

, a>0 , a≠1 , b>0 , p and q are real numbers, q≠0 , in particular for b=a we have

Formulations and proofs of properties

We proceed to the formulation and proof of the written properties of logarithms. All properties of logarithms are proven on the basis of the definition of the logarithm and the basic logarithmic identity that follows from it, as well as the properties of the degree.

Let's start with properties of the logarithm of one. Its formulation is as follows: the logarithm of unity is equal to zero, that is, log a 1=0 for any a>0, a≠1. The proof is not difficult: since a 0 =1 for any a satisfying the above conditions a>0 and a≠1, then the equality log a 1=0 to be proved follows immediately from the definition of the logarithm.

Let us give examples of the application of the considered property: log 3 1=0, log1=0 and .

Let's move on to the next property: the logarithm of a number equal to the base is equal to one, that is, log a a=1 for a>0, a≠1. Indeed, since a 1 =a for any a, then by definition of the logarithm log a a=1.

Examples of the use of this property of logarithms are the equalities log 5 5=1, log 5.6 5.6 and lne=1.

The logarithm of a power of a number equal to the base of the logarithm is equal to the exponent. This property of the logarithm corresponds to a formula of the form log a a p =p, where a>0, a≠1 and p – any real number. This property follows directly from the definition of the logarithm. Note that it allows you to immediately indicate the value of the logarithm, if it is possible to represent the number under the logarithm sign as a power of the base; we will talk more about this in the article calculating logarithms.

For example, log 2 2 7 =7, log10 -4 =-4 and .

Logarithm of the product of two positive numbers x and y is equal to the product of the logarithms of these numbers: log a (x y)=log a x+log a y, a>0 , a≠1 . Let us prove the property of the logarithm of a product. Due to the properties of the degree a log a x+log a y =a log a x ·a log a y, and since by the main logarithmic identity a log a x =x and a log a y =y, then a log a x ·a log a y =x· y. Thus, a log a x+log a y =x·y, from which, by the definition of a logarithm, the equality being proved follows.

Let's show examples of using the property of the logarithm of a product: log 5 (2 3)=log 5 2+log 5 3 and .

The property of the logarithm of a product can be generalized to the product of a finite number n of positive numbers x 1 , x 2 , …, x n as log a (x 1 · x 2 ·…·x n)= log a x 1 +log a x 2 +…+log a x n. This equality can be proven without problems using the method of mathematical induction.

For example, the natural logarithm of the product can be replaced by the sum of three natural logarithms of the numbers 4, e, and.

Logarithm of the quotient of two positive numbers x and y is equal to the difference between the logarithms of these numbers. The property of the logarithm of a quotient corresponds to a formula of the form , where a>0, a≠1, x and y are some positive numbers. The validity of this formula is proven as well as the formula for the logarithm of a product: since , then by definition of the logarithm .

Here is an example of using this property of the logarithm: .

Let's move on to property of the logarithm of the power. The logarithm of a degree is equal to the product of the exponent and the logarithm of the modulus of the base of this degree. Let us write this property of the logarithm of a power as a formula: log a b p =p·log a |b|, where a>0, a≠1, b and p are numbers such that the degree b p makes sense and b p >0.

First we prove this property for positive b. The basic logarithmic identity allows us to represent the number b as a log a b , then b p =(a log a b) p , and the resulting expression, due to the property of power, is equal to a p·log a b . So we come to the equality b p =a p·log a b, from which, by the definition of a logarithm, we conclude that log a b p =p·log a b.

It remains to prove this property for negative b. Here we note that the expression log a b p for negative b makes sense only for even exponents p (since the value of the degree b p must be greater than zero, otherwise the logarithm will not make sense), and in this case b p =|b| p. Then b p =|b| p =(a log a |b|) p =a p·log a |b| , from where log a b p =p·log a |b| .

For example, and ln(-3) 4 =4·ln|-3|=4·ln3 .

It follows from the previous property property of the logarithm from the root: the logarithm of the nth root is equal to the product of the fraction 1/n by the logarithm of the radical expression, that is, where a>0, a≠1, n is a natural number greater than one, b>0.

The proof is based on the equality (see definition of exponent with a fractional exponent), which is valid for any positive b, and the property of the logarithm of the exponent: .

Here is an example of using this property: .

Now let's prove formula for moving to a new logarithm base kind . To do this, it is enough to prove the validity of the equality log c b=log a b·log c a. The basic logarithmic identity allows us to represent the number b as a log a b , then log c b=log c a log a b . It remains to use the property of the logarithm of the degree: log c a log a b =log a b·log c a . This proves the equality log c b=log a b·log c a, which means the formula for transition to a new base of the logarithm is also proven .

Let's show a couple of examples of using this property of logarithms: and .

The formula for moving to a new base allows you to move on to working with logarithms that have a “convenient” base. For example, it can be used to change to natural or decimal logarithms so that you can calculate the value of a logarithm from a table of logarithms. The formula for moving to a new logarithm base also allows, in some cases, to find the value of a given logarithm when the values of some logarithms with other bases are known.

Often used special case formulas for transition to a new base of the logarithm with c=b of the form. This shows that log a b and log b a are mutually inverse numbers. For example, .

The formula is also often used, which is convenient for finding the values of logarithms. To confirm our words, we will show how it can be used to calculate the value of a logarithm of the form . We have . To prove the formula, it is enough to use the formula for moving to a new base of the logarithm a: .

It remains to prove the properties of comparison of logarithms.

Let's use the opposite method. Suppose that for a 1 >1, a 2 >1 and a 1 2 and for 0 1, log a 1 b≤log a 2 b is true. Based on the properties of logarithms, these inequalities can be rewritten as And respectively, and from them it follows that log b a 1 ≤log b a 2 and log b a 1 ≥log b a 2, respectively. Then, according to the properties of powers with the same bases, the equalities b log b a 1 ≥b log b a 2 and b log b a 1 ≥b log b a 2 must hold, that is, a 1 ≥a 2 . So we came to a contradiction to the condition a 1 2. This completes the proof.

Basic properties of logarithms

Materials for the lesson
Download all formulas

Logarithms, like any numbers, can be added, subtracted and transformed in every way. But since logarithms are not exactly ordinary numbers, there are rules here, which are called main properties.

You definitely need to know these rules - without them not a single serious logarithmic problem can be solved. In addition, there are very few of them - you can learn everything in one day. So let's get started.

Adding and subtracting logarithms

Consider two logarithms with the same bases: log a x and log a y. Then they can be added and subtracted, and:

So, the sum of logarithms is equal to the logarithm of the product, and the difference is equal to the logarithm of the quotient. Please note: the key point here is identical grounds. If the reasons are different, these rules do not work!

These formulas will help you calculate a logarithmic expression even when its individual parts are not considered (see the lesson “What is a logarithm”). Take a look at the examples and see:

Task. Find the value of the expression: log 6 4 + log 6 9.

Since logarithms have the same bases, we use the sum formula:
log 6 4 + log 6 9 = log 6 (4 9) = log 6 36 = 2.

Task. Find the value of the expression: log 2 48 − log 2 3.

The bases are the same, we use the difference formula:
log 2 48 − log 2 3 = log 2 (48: 3) = log 2 16 = 4.

Task. Find the value of the expression: log 3 135 − log 3 5.

Again the bases are the same, so we have:
log 3 135 − log 3 5 = log 3 (135: 5) = log 3 27 = 3.

As you can see, the original expressions are made up of “bad” logarithms, which are not calculated separately. But after the transformations, completely normal numbers are obtained. Many tests are based on this fact. Yes, test-like expressions are offered in all seriousness (sometimes with virtually no changes) on the Unified State Examination.

Extracting the exponent from the logarithm

log a x n = n · log a x ;

It is easy to see that the last rule follows the first two. But it’s better to remember it anyway - in some cases it will significantly reduce the amount of calculations.

Of course, all these rules make sense if the ODZ of the logarithm is observed: a > 0, a ≠ 1, x > 0. And one more thing: learn to apply all formulas not only from left to right, but also vice versa, i.e. You can enter the numbers before the logarithm sign into the logarithm itself. This is what is most often required.

Task. Find the value of the expression: log 7 49 6 .

Let's get rid of the degree in the argument using the first formula:
log 7 49 6 = 6 log 7 49 = 6 2 = 12

Task. Find the meaning of the expression:

[Caption for the picture]

Note that the denominator contains a logarithm, the base and argument of which are exact powers: 16 = 2 4 ; 49 = 7 2. We have:

[Caption for the picture]

Transition to a new foundation

Formulas for transition to a new foundation come to the rescue. Let us formulate them in the form of a theorem:

Let the logarithm log a x be given. Then for any number c such that c > 0 and c ≠ 1, the equality is true:

[Caption for the picture]

In particular, if we set c = x, we get:

[Caption for the picture]

These formulas are rarely found in ordinary numerical expressions. It is possible to evaluate how convenient they are only when solving logarithmic equations and inequalities.

However, there are problems that cannot be solved at all except by moving to a new foundation. Let's look at a couple of these:

Task. Find the value of the expression: log 5 16 log 2 25.

Note that the arguments of both logarithms contain exact powers. Let's take out the indicators: log 5 16 = log 5 2 4 = 4log 5 2; log 2 25 = log 2 5 2 = 2log 2 5;

Now let’s “reverse” the second logarithm:

[Caption for the picture]

Since the product does not change when rearranging factors, we calmly multiplied four and two, and then dealt with logarithms.

Task. Find the value of the expression: log 9 100 lg 3.

The base and argument of the first logarithm are exact powers. Let's write this down and get rid of the indicators:

[Caption for the picture]

Now let's get rid of the decimal logarithm by moving to a new base:

[Caption for the picture]

Basic logarithmic identity

Often in the solution process it is necessary to represent a number as a logarithm to a given base. In this case, the following formulas will help us:

n = log a a n
In the first case, the number n becomes the exponent in the argument. The number n can be absolutely anything, because it is just a logarithm value.

The second formula is actually a paraphrased definition. That’s what it’s called: the basic logarithmic identity.

In fact, what happens if the number b is raised to such a power that the number b to this power gives the number a? That's right: the result is the same number a. Read this paragraph carefully again - many people get stuck on it.

Like formulas for moving to a new base, the basic logarithmic identity is sometimes the only possible solution.

[Caption for the picture]

Note that log 25 64 = log 5 8 - we simply took the square from the base and argument of the logarithm. Taking into account the rules for multiplying powers with the same base, we get:

[Caption for the picture]

If anyone doesn’t know, this was a real task from the Unified State Exam :)

Logarithmic unit and logarithmic zero

In conclusion, I will give two identities that can hardly be called properties - rather, they are consequences of the definition of the logarithm. They constantly appear in problems and, surprisingly, create problems even for “advanced” students.
1. log a a = 1 is a logarithmic unit. Remember once and for all: the logarithm to any base a of that base itself is equal to one.
2. log a 1 = 0 is logarithmic zero. The base a can be anything, but if the argument contains one, the logarithm is equal to zero! Because a 0 = 1 is a direct consequence of the definition.
That's all the properties. Be sure to practice putting them into practice! Download the cheat sheet at the beginning of the lesson, print it out, and solve the problems.

Logarithm. Properties of the logarithm (addition and subtraction).

Properties of the logarithm follow from its definition. And so the logarithm of the number b based on A is defined as the exponent to which a number must be raised a to get the number b(logarithm exists only for positive numbers).

From this formulation it follows that the calculation x=log a b, is equivalent to solving the equation a x =b. For example, log 2 8 = 3 because 8 = 2 3 . The formulation of the logarithm makes it possible to justify that if b=a c, then the logarithm of the number b based on a equals With. It is also clear that the topic of logarithms is closely related to the topic of powers.

With logarithms, as with any numbers, you can do operations of addition, subtraction and transform in every possible way. But due to the fact that logarithms are not entirely ordinary numbers, their own special rules apply here, which are called main properties.

Adding and subtracting logarithms.

Let's take two logarithms with the same bases: log a x And log a y. Then it is possible to perform addition and subtraction operations:

As we see, sum of logarithms equals the logarithm of the product, and difference logarithms- logarithm of the quotient. Moreover, this is true if the numbers A, X And at positive and a ≠ 1.

It is important to note that the main aspect in these formulas are the same bases. If the grounds are different, these rules do not apply!

The rules for adding and subtracting logarithms with the same bases are read not only from left to right, but also vice versa. As a result, we have the theorems for the logarithm of the product and the logarithm of the quotient.

Logarithm of the product two positive numbers is equal to the sum of their logarithms ; paraphrasing this theorem we get the following if the numbers A, x And at positive and a ≠ 1, That:

Logarithm of the quotient two positive numbers is equal to the difference between the logarithms of the dividend and the divisor. To put it another way, if the numbers A, X And at positive and a ≠ 1, That:

Let us apply the above theorems to solve examples:

If the numbers x And at are negative, then product logarithm formula becomes meaningless. Thus, it is forbidden to write:

since the expressions log 2 (-8) and log 2 (-4) are not defined at all (logarithmic function at= log 2 X defined only for positive values argument X).

Product theorem applicable not only for two, but also for an unlimited number of factors. This means that for every natural k and any positive numbers x 1 , x 2 , . . . ,x n there is an identity:

From logarithm quotient theorem One more property of the logarithm can be obtained. It is common knowledge that log a 1= 0, therefore

This means there is an equality:

Logarithms of two mutually reciprocal numbers for the same reason will differ from each other solely by sign. So:

Logarithm. Properties of logarithms

Logarithm. Properties of logarithms

Let's consider equality. Let us know the values of and and we want to find the value of .

That is, we are looking for the exponent by which we need to cock it to get .

Let a variable can take on any real value, then the following restrictions are imposed on the variables: o" title="a>o"/> , 1″ title="a1″/>, 0″ title="b>0″/>

If we know the values of and , and we are faced with the task of finding the unknown, then for this purpose a mathematical operation is introduced, which is called logarithm.

To find the value we take logarithm of a number By basis :

The logarithm of a number to its base is the exponent to which it must be raised to get .

That is basic logarithmic identity:

o» title=»a>o»/> , 1″ title=»a1″/>, 0″ title=»b>0″/>

is essentially a mathematical notation definitions of logarithm.

The mathematical operation of logarithm is the inverse of the operation of exponentiation, so properties of logarithms are closely related to the properties of degree.

Let's list the main properties of logarithms:

(o" title="a>o"/> , 1″ title=»a1″/>, 0″ title=»b>0″/>, 0,

d>0″/>, 1″ title=”d1″/>

4.

5.

The following group of properties allows you to represent the exponent of an expression under the sign of the logarithm, or standing at the base of the logarithm in the form of a coefficient in front of the sign of the logarithm:

6.

7.

8.

9.

The next group of formulas allows you to move from a logarithm with a given base to a logarithm with an arbitrary base, and is called formulas for transition to a new base:

10.

12. (corollary from property 11)

The following three properties are not well known, but they are often used when solving logarithmic equations, or when simplifying expressions containing logarithms:

13.

14.

15.

Special cases:

— decimal logarithm

— natural logarithm

When simplifying expressions containing logarithms, a general approach is used:

1. Introducing decimals in the form of ordinary ones.

2. Mixed numbers represented as improper fractions.

3. We decompose the numbers at the base of the logarithm and under the sign of the logarithm into simple factors.

4. We try to reduce all logarithms to the same base.

5. Apply the properties of logarithms.

Let's look at examples of simplifying expressions containing logarithms.

Example 1.

Calculate:

Let's simplify all exponents: our task is to reduce them to logarithms, the base of which is the same number as the base of the exponent.

==(by property 7)=(by property 6) =

Let's substitute the indicators that we got into the original expression. We get:

Answer: 5.25

Example 2. Calculate:

Let's reduce all logarithms to base 6 (in this case, the logarithms from the denominator of the fraction will “migrate” to the numerator):

Let's decompose the numbers under the logarithm sign into simple factors:

Let's apply properties 4 and 6:

Let's introduce the replacement

We get:

Answer: 1

Logarithm . Basic logarithmic identity.

Properties of logarithms. Decimal logarithm. Natural logarithm.

Logarithm positive number N to base (b > 0, b 1) is the exponent x to which b must be raised to get N .

This entry is equivalent to the following: b x = N .

Examples: log 3 81 = 4, since 3 4 = 81;

log 1/3 27 = – 3, since (1/3) - 3 = 3 3 = 27.

The above definition of logarithm can be written as an identity:

Basic properties of logarithms.

2) log 1 = 0, since b 0 = 1 .

3) The logarithm of the product is equal to the sum of the logarithms of the factors:

4) The logarithm of the quotient is equal to the difference between the logarithms of the dividend and the divisor:

5) The logarithm of a power is equal to the product of the exponent and the logarithm of its base:

The consequence of this property is the following: logarithm of the root equal to the logarithm of the radical number divided by the power of the root:

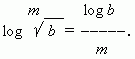
6) If the base of the logarithm is a degree, then the value the inverse of the exponent can be taken out of the log rhyme sign:

The last two properties can be combined into one:

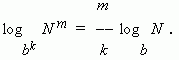
7) Transition modulus formula (i.e. transition from one logarithm base to another base):

In the special case when N=a we have:

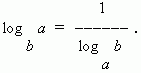
Decimal logarithm called base logarithm 10. It is designated lg, i.e. log 10 N= log N. Logarithms of numbers 10, 100, 1000, . p are 1, 2, 3, …, respectively, i.e. have so many positive

units, how many zeros are there in a logarithmic number after one. Logarithms of numbers 0.1, 0.01, 0.001, . p are respectively –1, –2, –3, …, i.e. have as many negative ones as there are zeros in the logarithmic number before one (including zero integers). The logarithms of other numbers have a fractional part called mantissa. Whole part the logarithm is called characteristic. For practical use, decimal logarithms are most convenient.

Natural logarithm called base logarithm e. It is denoted by ln, i.e. log e N= log N. Number e is irrational, its approximate value is 2.718281828. It is the limit to which the number tends (1 + 1 / n) n with unlimited increase n(cm. first wonderful limit on the "Number Sequence Limits" page).
Strange as it may seem, natural logarithms turned out to be very convenient when carrying out various kinds operations related to function analysis. Calculating logarithms to the base e carried out much faster than for any other reason.

What is needed today to adopt a child in Russia? Adoption in Russia, in addition to a responsible personal decision, involves a number of procedures for state verification of candidates. Tough selection for preparatory stage contributes to more […]
Free information on TIN or OGRN from the tax register throughout Russia - online Information on state registration can be obtained on the Unified Tax Services Portal legal entities, individual entrepreneurs, […]
Penalty for driving without documents ( driver license, insurance, STS) Sometimes, due to forgetfulness, drivers get behind the wheel without a driver's license and receive a fine for driving without documents. We would like to remind you that a car enthusiast is required to have […]
Flowers for men. What flowers can you give a man? What flowers can you give a man? There are not many “male” flowers, but there are some that are given to men. A small flower list in front of you: Chrysanthemums. Roses. Carnations. […]
A memo is special form document that is used in internal environment enterprises and serves for quick solution current production problems. Typically this document is drawn up for the purpose of introducing some […]
When and how to receive the funded part of your pension from Sberbank? Sberbank is a partner bank of the state pension fund. Based on this, citizens who registered for a funded pension could transfer the funded part […]
Child benefits in Ulyanovsk and the Ulyanovsk region in 2018 In addition, programs approved by federal legislation. Let's look at who can count on what benefits. How regional authorities […]
Detailed Guide how to draw up a power of attorney to represent interests individual in court In a civil or arbitration claim, in an administrative or criminal case, the interests of both the plaintiff and the defendant may be represented by an attorney: […]